Boltzmann factor and Maxwell-and-Boltzmann disbribution
www.youtube.com/watch?v=mERpRoZeLpQ
==================================================
$$$S=k \ln\Omega$$$
S is entropy
k is Boltzmann constant value
$$$\Omega$$$ is numeric which represents state of system
In other words, $$$\Omega$$$ is number of possible states which system can have
==================================================
Above eqation is notation of Entropy in terms of statistics
==================================================
When you think of Entropy in terms of thermodynamics
$$$S=\dfrac{Q}{T} $$$
Q is heat energy
T is absolute temperature
==================================================
You can write above ones without S
$$$k \ln\Omega = \dfrac{Q}{T}$$$
Then,
$$$\Omega = e^{\frac{Q}{kT}}$$$
In physics, $$$\dfrac{1}{kT}$$$ is notated by $$$\beta$$$
$$$\dfrac{1}{kT}=\beta$$$
==================================================
From now on, to think of generalization
you'll consider Q as energy than heat energy
==================================================
$$$\dfrac{C}{\Omega} = P$$$
$$$\Omega$$$ is "all" possible number on state which system can have
C is "specific" number for energy
Then, it means probability P
Not clear
$$$\dfrac{C}{\Omega} = P = Ce^{-\beta E}$$$
You'll just write $$$C$$$ as $$$\dfrac{1}{Z}$$$
$$$= \dfrac{1}{Z} e^{-\beta E}$$$
You know following characteristic of probability
$$$\sum P =1$$$
==================================================
From characteristic of probability, you can wrtie as
$$$\sum P = 1 = \dfrac{1}{Z} \sum e^{-\beta E} $$$
==================================================
$$$Z= \sum e^{-\beta E}$$$
Z is called distributing function
$$$e^{-\beta E}$$$ is called Boltzmann factor
==================================================
Maxwell-Boltzmann disbribution is velocity disbribution of molecules of air
Molecules of air will move in heat
Then, what's velocity distribution in that movement?
You can't measure velocity of all molecules of air
So, you need to use statistics
==================================================
You will ignore interaction between molecules of air
which means you ignore potential energy of molecules of air
which means you only consider kinetic energy of molecules of air into equation
==================================================
Kinetic energy as non-relative way is $$$\dfrac{1}{2}mv^2$$$
Probability function with respect to velocity v is as following
$$$P(v) =
\dfrac{1}{Z} e ^{-\frac{1}{2}\beta mv^2}$$$
==================================================
v can be written sum of square of v in each axis
$$$v^2 = v_x^2 +v_y^2 +v_z^2 $$$
==================================================
Image sphere in 3D space
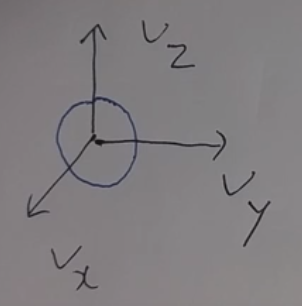 $$$dv_x dv_y dv_z = 4\pi v^2 dv$$$
$$$dv_x$$$ is small v in x axis
==================================================
$$$\int_{-\infty}^{\infty} x^2 e^{-\lambda x^2} dx = \dfrac{\sqrt{\pi}}{2} \lambda^{-\frac{3}{2}}$$$
$$$x^2 e^{-\lambda x^2}$$$ is symmetric in y axis
so you can write
$$$\int_{0}^{\infty} x^2 e^{-\lambda x^2} dx = \dfrac{\sqrt{\pi}}{4} \lambda^{-\frac{3}{2}}$$$
==================================================
Apply above notation into kinetic energy
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx $$$
After perform intergration, you get following
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx =
4\pi A \times \dfrac{\sqrt{\pi}}{4}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}}$$$
According to characteristic of probability,
result should be 1
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx
= 4\pi A \times \dfrac{\sqrt{\pi}}{4}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}}
= 1$$$
==================================================
If you delete 2 of 4, then
$$$\pi A \times \sqrt{\pi}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}} = 1$$$
$$$A = \dfrac{1}{\pi \sqrt{\pi}} \left( \dfrac{\beta m}{2} \right)^{\frac{3}{2}}$$$
You already know $$$\pi \sqrt{\pi} = \pi^{\frac{2}{3}}$$$
$$$= \left( \dfrac{\beta m}{2\pi} \right)^{\frac{3}{2}}$$$
Constance value A will be used for nomalization
==================================================
In Maxwell-Boltzmann distribution,
velocity disbribution function will be
$$$\rho(v) = \left( \dfrac{\beta m}{2\pi} \right)^{\frac{3}{2}}
4\pi v^2
e^{-\frac{1}{2}\beta m v^2}$$$
==================================================
Since you have velocity disbribution function
you can get avaerga of velocity by performing intergration
In probablistic math, when you calculate average
you multiply variable by probability function
then sum them all
You use same way for this
$$$\big< v \big> =
\int_{0}^{\infty} v \times \rho(v) dv$$$
$$$\big< v \big>$$$ is average of velocity
==================================================
$$$k=\dfrac{R}{N_A}$$$
$$$k$$$ is Boltzmann constant
$$$R$$$ is gas constant ($$$10^{23}$$$)
$$$N_A$$$ is avogadro number ($$$6.02$$$)
==================================================
Let's just try this
$$$M=m\times N_A$$$
==================================================
And by using all of terms
after you perform intergration
you get average of velocity as follow
$$$\big<v\big> = \sqrt{\dfrac{8RT}{\pi M}}$$$
==================================================
$$$v_{rms}$$$ is root mean square of v?
$$$v_{rms} =
\left( \int_0^{-\infty} v^2 \rho(v) dv \right)^{\frac{1}{2}}$$$
Then you finally get
$$$= \sqrt{\dfrac{3RT}{M}}$$$
$$$dv_x dv_y dv_z = 4\pi v^2 dv$$$
$$$dv_x$$$ is small v in x axis
==================================================
$$$\int_{-\infty}^{\infty} x^2 e^{-\lambda x^2} dx = \dfrac{\sqrt{\pi}}{2} \lambda^{-\frac{3}{2}}$$$
$$$x^2 e^{-\lambda x^2}$$$ is symmetric in y axis
so you can write
$$$\int_{0}^{\infty} x^2 e^{-\lambda x^2} dx = \dfrac{\sqrt{\pi}}{4} \lambda^{-\frac{3}{2}}$$$
==================================================
Apply above notation into kinetic energy
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx $$$
After perform intergration, you get following
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx =
4\pi A \times \dfrac{\sqrt{\pi}}{4}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}}$$$
According to characteristic of probability,
result should be 1
$$$\int_{0}^{\infty} Ae^{-\frac{1}{2}\beta mv^2} 4\pi v^2 dx
= 4\pi A \times \dfrac{\sqrt{\pi}}{4}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}}
= 1$$$
==================================================
If you delete 2 of 4, then
$$$\pi A \times \sqrt{\pi}\left( \dfrac{\beta m}{2} \right)^{-\frac{3}{2}} = 1$$$
$$$A = \dfrac{1}{\pi \sqrt{\pi}} \left( \dfrac{\beta m}{2} \right)^{\frac{3}{2}}$$$
You already know $$$\pi \sqrt{\pi} = \pi^{\frac{2}{3}}$$$
$$$= \left( \dfrac{\beta m}{2\pi} \right)^{\frac{3}{2}}$$$
Constance value A will be used for nomalization
==================================================
In Maxwell-Boltzmann distribution,
velocity disbribution function will be
$$$\rho(v) = \left( \dfrac{\beta m}{2\pi} \right)^{\frac{3}{2}}
4\pi v^2
e^{-\frac{1}{2}\beta m v^2}$$$
==================================================
Since you have velocity disbribution function
you can get avaerga of velocity by performing intergration
In probablistic math, when you calculate average
you multiply variable by probability function
then sum them all
You use same way for this
$$$\big< v \big> =
\int_{0}^{\infty} v \times \rho(v) dv$$$
$$$\big< v \big>$$$ is average of velocity
==================================================
$$$k=\dfrac{R}{N_A}$$$
$$$k$$$ is Boltzmann constant
$$$R$$$ is gas constant ($$$10^{23}$$$)
$$$N_A$$$ is avogadro number ($$$6.02$$$)
==================================================
Let's just try this
$$$M=m\times N_A$$$
==================================================
And by using all of terms
after you perform intergration
you get average of velocity as follow
$$$\big<v\big> = \sqrt{\dfrac{8RT}{\pi M}}$$$
==================================================
$$$v_{rms}$$$ is root mean square of v?
$$$v_{rms} =
\left( \int_0^{-\infty} v^2 \rho(v) dv \right)^{\frac{1}{2}}$$$
Then you finally get
$$$= \sqrt{\dfrac{3RT}{M}}$$$
